This Calculator Will Help You To calculate The Transmission and Reflection of Coefficient of Light At Non-normal Incidence of Uniaxial Crystal (Single Surface)

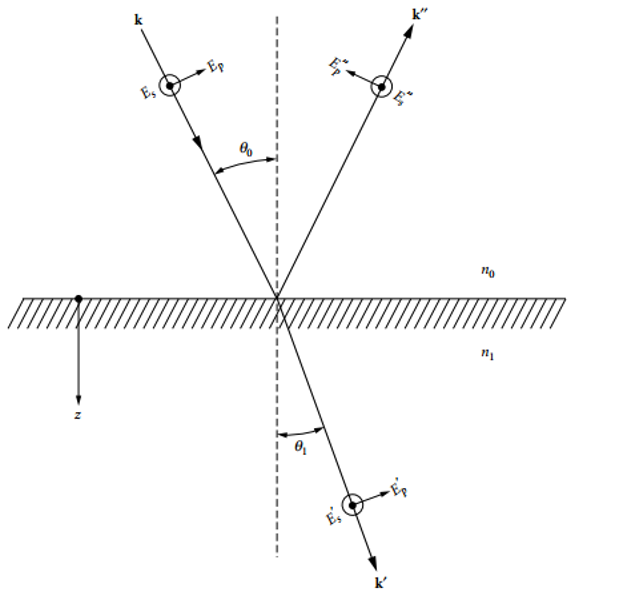
www.simtrum.com

$n_1$sin$\theta_1$=$n_0$sin$\theta_0$

$T_s$=$\frac{ sin 2 \theta_0 sin 2 \theta_1}{sin^2(\theta_0 + \theta_1)}$
$R_s$=$\frac{ sin^2(\theta_0 - \theta_1)}{sin^2(\theta_0 + \theta_1)}$

$T_p$=$\frac{ sin 2 \theta_0 sin 2 \theta_1}{sin^2(\theta_0 + \theta_1) cos^2(\theta_0 - \theta_1)}$
$R_p$=$\frac{ tan^2(\theta_0 - \theta_1)}{ tan^2(\theta_0 + \theta_1)}$

Incident angle of the light $\theta_0$; Refraction angle of the light $\theta_1$; Transmission Coefficient with polarization is out of the paper $T_s$; Transmission Coefficient with polarization is in the plane of the paper $T_p$; Reflection Coefficient with polarization is out of the paper $R_s$; Reflection Coefficient with polarization is in the plane of the paper $R_p$


Input
Incident Angle, $\theta_0$:
degree (°)
$n_0$:
$n_1$:





Output
$T_s$:
$T_p$:
$R_s$:
$R_p$: